PWPB95-D3: 50% HF exchange + 50% Re-Fit PW91 GGA exchange + 73.1% Re-Fit B95 meta-GGA correlation + 26.9% OS MP2 correlation with DFT-D3(0) tail 309 5.3.3 Correlation Functionals References and Further Reading 5.3.5 Specialized Functionals. Density functional theory (DFT) is the standard formalism to study the electronic structure of matter at the atomic scale. In Kohn–Sham DFT simulations, the balance between accuracy and computational cost depends on the choice of exchange and correlation functional, which only exists in approximate form. Nature of problem: Evaluation of the exchange–correlation energy functional and its derivatives. This is a fundamental part of any atomic, molecular, or solid-state code that uses density-functional theory. Solution method: The values of the energy functional and its derivatives are given in a real grid of mesh points.

ADS! Download JAMB CBT Software Now for FREE!

Exchange Correlation Functional In Dft

Towards A New Exchange-Correlation Density Functional for More Accurate Band Gap Predictions.

Abstract

Density-Functional Theory (DFT) offers a simplification to electronic structure prob- lems by using the electron density instead of the wave-function.

Unlike the wave- function which is a function of 3N variables (excluding spin) for an N -electron system, the density depends only on three variables, irrespective of the number of electrons in the system.

While DFT, in principle, gives an accurate description of ground-state properties, practical applications of DFT are based on approximations to the so-called exchange-correlation (xc) potential.

The exchange-correlation po- tential describes the effects of the Pauli exclusion principle and the electron-electron Coulomb repulsion beyond a purely electrostatic interaction of the electrons.

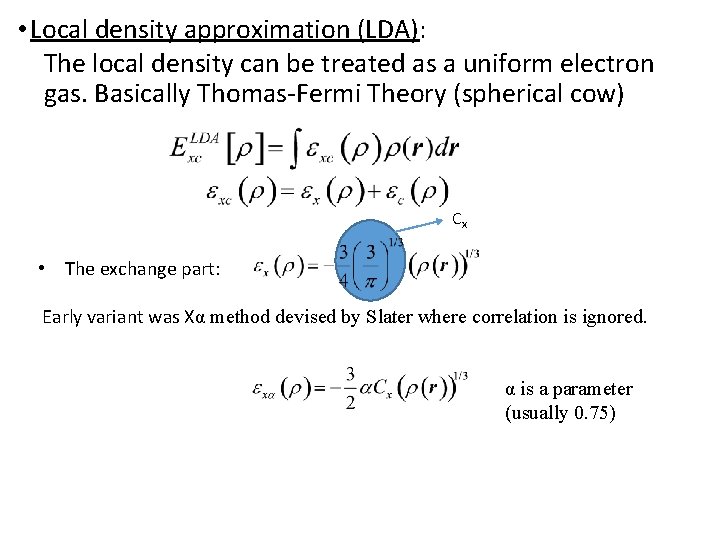
A common description of exchange-correlation functional is the so-called local den- sity approximation (LDA) which locally substitutes the exchange-correlation energy density of an inhomogeneous system by that of an electron gas evaluated at that local density.

While many ground state properties (such as lattice constants and bulk moduli) are well described in the LDA, the band gap is underestimated by as much as 50% in LDA compared to experiments.

In this thesis, we focus on the development of an exchange-correlation functional with adjustable parameters which can give more accurate band gap energies.

This functional is based on the xc potential derived in 1988 from a tight-binding approx- imation by Hanke and Sham (HS).

Our contribution consists in expressing the HS potential in terms of the electron density and its gradient.

Table Of Contents

Abstract i

Acknowledgments ii

Dedication iv

Contents vi

• Introduction 1
  • Successes and Failures of DFT….2
  • The Band Gap Problem…………..3
  • Atomic Units……………….. 4
• Theoretical Background 6
  • Electronic Structure Problem………… 6
  • Wave-Function Based Methods………………. 9
    • The Hartree-Fock Formalism…………… 9
    • Correlated Methods Beyond Hartree-Fock………… 13
  • Density-Functional Thoery…………. 13
    • The Thomas-Fermi (TF) Model…………. 14
    • The Hohenberg-Kohn (HK) Theorem…..15
  • The Kohn-Sham (KS) Scheme……. 17
  • Interpretation of Kohn-Sham energies………20
  • Failure of DFT for Band Gap Energies……..20

• Past Work on Correcting DFT Band Gap Energies….22

• Functional Development 25
  • Exchange-Correlation Functionals………………. 25
    • The Local-Density Approximation (LDA)……….. 26
    • The Generalized-Gradient Approximation (GGA)…. 27
    • Meta-GGA (mGGA)……………… 28
    • Hybrid Schemes………………….. 29
  • A New Density Functional…………. 30
    • Xα-Method……………….30
    • The Corresponding xc Energy E_xc……… 32
  • Methods 35
    • Basis Sets……………………………………. 35
      • Plane Waves…………. 36
      • Pseudo-potentials……………….. 37
    • Methodology………………………… 40
  • Results, Discussion and Conclusion 42
    • Results…………… 42
    • Discussion…………………… 48
    • Conclusion and Perspective……………… 48
  • Hanke-Sham (HS) xc Potential 49
    • Green’s Function and Self-Energy Operator…………. 49
    • A Tight-Binding (TB) Model for the Self-Energy (Σ_xc)……….. 51
    • A v_xc for Insulators and Semiconductors………….. 54

Bibliography 57

Introduction

Background Of Study

Density-Functional Theory (DFT) is one of the most popular and successful Quan- tum Mechanics (QM) approach for large systems.

It is a widely used methods for “ab initio” calculations of the structure of atoms, molecules, crystals, surfaces and their interactions.

It is nowadays routinely applied for calculating e.g., the binding energy of molecules in chemistry and the band structure of solids in physics.

First application relevant for fields traditionally considered more distant from quantum mechanics, such as biology and mineralogy are beginning to appear.

Superconduc- tivity, atoms in the focus of strong laser pulses, relativistic effects in heavy elements and in atomic nuclei, classical liquids, and magnetic properties of alloys have been studied with DFT.

What Is Exchange Correlation Functional

Bibliography

Hanke, L.J. Sham Phys. Rev. B 38, 13361 (1988)

Capelle, A bird’s-eye view of density-functional theory, http://arxiv.org/abs/cond-mat/0211443.

Fermi E (1926) Z Phys 36:902.

Dirac PAM (1930) Proc Cam Phil Soc 26:376.

Baroni and R. Resta, Phys. Rev B 33, 7017 (1986).

Hybertson and S. Louie, Phys. Rev. B 34, 5390 (1986).

Join Over 3,500 000+ Readers Online Now!

=>FOLLOW US ON INSTAGRAM | FACEBOOK & TWITTER FOR LATEST UPDATES

ADS: KNOCK-OFF DIABETES IN JUST 60 DAYS! - ORDER YOURS HERE